Pythagoras Growing Post

PART 1.

Describe what a Pythagorean Triple is and use your perfect square chart from 1 squared to 10 squared to find another one other than 3,4,5. (Note: You need a picture, not simply text.)

What is a Pythagorean Triple ?

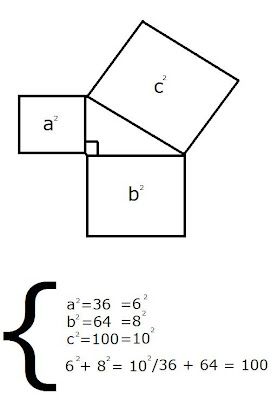
A pythagorean triple is an equation of a² + b² = c ² . A and b are the the legs of a right angled triangle and when the lengths of the legs are added together they equal the hypotenuse which is c. İt uses only 3 perfect square numbers for this question
a² + b² = c²

An example of a pythagorean triple using the perfect square chart:

A² + B² = C²

PART 2.

Using embeddable web 2.0, describe how to find the missing side of a Right Triangle using the Pythagorean Theorem, show how to solve both for a missing leg and the hypotenuse (note: you may use numerical examples ie. a=4 b=6 c=?)

BubbleShare: Share photos - Powered by BubbleShare


PART 3 :
Explain how to solve a Pythagoras word problem. Use one of the examples we covered in class (Worksheet A, B, LeFrog or Bonus Problem).

Lefrog stands 1.5 meters from a wall where a fly is 2 meters up. if Lefrog's tongue comes out of his mouth 70 cm from the ground, how far does his tongue have to stretch to eat the fly ?

This would be the information they gave us in the question:
[ Lefrog stands 1.5 meters from the wall
[ a fly is 2 meters up the wall
[ his tongue comes out 70cm from the ground

It would then look like this . .

The formula for this would be:
a² + b² = c²

You would then fill in the a², b², or c² numbers that you know into the formula, and in the question they only gave us the a and b sides.
1.3m² + 1.5m² = c²

Now you have to square the numbers off by multiplying the base numbers by itself.
1.3m x 1.3m = 1.69m
1.5m x 1.5m = 2.25m

The next part is the area of the squares sides above, so when you add these two areas together it will equal the area of c².
1.69m + 2.25m = c²

So now you add these two together to get the answer for c².
1.69m + 2.25m = 3.94m

Since now we know what c² is you have to find the square root of it by using a calculator. You put in 3.94 and then press the square root button and now you have the the answer to the question.
c² = 3.94m
square root of c² = 1.98m

Lefrogs tongue has to stretch 1.98m to eat the fly.

PART 4.

Now that you have seen many Pythagorean problems, create your own word problem.

Billy-bob is playing basketball at the court, the basket is 8 meters high. Billy-bob is at the foul line which is 10 meters away from the basket. How high does Billy-bob have to shoot the ball to get a basket?